What is Vibration

"Vibration" is the term used to describe a continuing periodic change in the magnitude of a displacement with respect to a specified central reference. The periodic motion may range from the simple to-and-fro oscillations of a pendulum, through the more complicated vibrations of a steel plate when struck with a hammer, to the extremely complicated vibrations of large structures such as an automobile on a rough road. Vibrations are also experienced by atoms, molecules, and nuclei.

A mechanical system must possess the properties of mass and stiffness or their equivalents in order to be capable of self-supported free vibration. Stiffness implies that an alteration in the normal configuration of the system will result in a restoring force tending to return it to this configuration. Mass or inertia implies that the velocity imparted to the system in being restored to its normal configuration will cause it to overshoot this configuration. It's in consequence of the interplay of mass and stiffness that periodic vibrations in mechanical systems are possible.

Mechanical vibration is the term used to describe the continuing periodic motion of a solid body at any frequency. When the rate of vibration of the solid body ranges between 20 and 20,000 hertz (Hz), it may also be referred to as an acoustic vibration, for if these vibrations are transmitted to a human ear they will produce the sensation of sound. The vibration of such a solid body in contact with a fluid medium such as air or water induces the molecules of the medium to vibrate in a similar fashion and thereby transmit energy in the form of an acoustic wave. Finally, when such an acoustic wave impinges on a material body, it forces the latter into a similar acoustic vibration. In the case of the human ear it produces the sensation of sound.

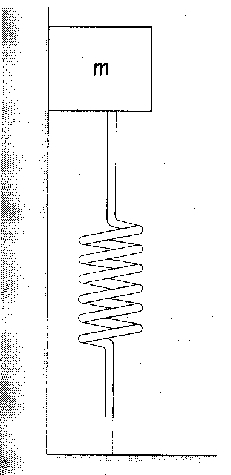
Fig. 1. Simple oscillator.

Systems with one degree of freedom are those for which one space coordinate alone is sufficient to specify the system's displacement from its normal configuration. An idealized example known as a simple oscillator consists of a point mass m fastened tone end of a massless spring and constrained to move back and forth in a line about its undisturbed position (Fig. 1, above). Although no actual acoustic vibrator is identical with this idealized example, the actual behavior of many vibrating systems when vibrating at low frequencies is similar and may be specified by giving values of a single space coordinate. (Read more about Harmonic Oscillators)

When the restoring force of the spring of a simple oscillator on its mass is directly proportional to the displacement of the latter from its normal position, the system vibrates in a sinusoidal manner called simple harmonic motion. This motion is identical with the projection of uniform circular motion on a diameter of a circle.

When two simple vibrating systems are interconnected by a flexible connection, the combined system has two degrees of freedom (Fig. 2). Such a system has two normal modes of vibration of two frequencies. Both of these frequencies differ from be respective natural frequencies of the individual uncoupled oscillators.

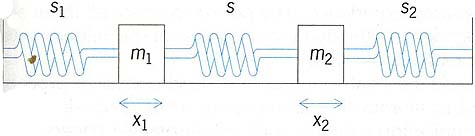
Fig. 2. Simple oscillator with two degrees of freedom. Masses m₁ and m₂, with displacements x₁ and x₂, are connected by springs s₁, s, and s₂.

A vibrating system is said to have several degrees of freedom many space coordinates are required to describe its motion. One example is n masses m₁, m₂, ..., m_n constrained to move in a line and interconnected by (n - 1) coupling springs with additional terminal springs leading from m₁ and m_n to rigid supports. This system has n normal modes of vibration, each of a distinct frequency.