Author: Peter W. Mitchell
Fans of straight-line tracking tonearms say that their favorite tracks better because its motion mimics the cutters head's movement, but a close analysis suggests that the chief virtue of radial-tracking tonearms is their low mass, not their straight-line tracking.

above: Dual ULM 741 turntable (ca. 1981)
It used to be that the radial tracking tonearm--which rides on a straight rail instead of pivoting in an arc from a base at one corner of the turntable was a complex and delicate mechanical arrangement of bearings, cams, motors, and leaf switches sensing any deviation of the arm from the perpendicular. It was costly, unreliable, and suitable only for the most devoted and finicky audiophiles. But lately the resources of contemporary electronics have been focused on the concept, and the result is a new generation of radials which are relatively inexpensive to manufacture and not at all fussy to operate. No longer an exotic luxury, the radial arm is now a fully practical alternative to the conventional pivoted tonearm.
When few radial arms were being made and sold, it didn't matter much whether claims for the inherent superiority of radial-tracking designs were really valid. But now, with radial arms competing vigorously for the turntable buyer's dollar, these claims are of interest to a great many audiophiles.
Consider, for example, the following language in a typical recent advertisement: "Foremost is the linear tracking mechanism itself. Much superior to ordinary pivoted tonearms in that it plays your records in the same straight line across the disc as the cutting head which made the original master recording. Tracking errors and unbalanced side forces are virtually nonexistent. The result is a dramatic decrease in distortion and false coloration. And a strikingly audible improvement in stereo definition and real-life presence." Putting aside the ad writer's customary exaggerations, is there really a solid physical basis for such claims? Do radial arms have a natural superiority arising from their unique mode of operation? Or are they just another gimmick? Neither. Radials are not just a gimmick. But they are not automatically better than pivoted tonearms. To put them in a realistic perspective and help you decide what tonearm is best for you, I'm going to analyze the differences between radial and pivoted arms, and we'll play detective-trying to identify why some tonearms really do sound better than others.
The claims for radial-arm superiority can be boiled down to three basic virtues: Perfect tangency, no skating force, and low arm mass. We will examine each of these in turn.
Tangential Tracking
The original motive for developing the radial arm was the idea that it could mimic the motion of the cutting lathe, holding the cartridge precisely tangent to the groove while moving it along the radius from the edge of the record toward its center. Logically, this seems like it would be the ideal way, the only really "right" way, for a tone arm to operate. But the real world is not quite that simple.
If records were cut with constant pitch (i.e. with a constant spacing between successive spirals of the groove), then it would be easy to drive the tonearm inward at exactly the right speed to maintain the cartridge tangent to the groove. But in fact modern records are cut with variable pitch; the groove spacing increases to accommodate loud passages, especially those with a lot of low-frequency energy, and decreases when the music is soft. Thus, the massive arm on the record cutting lathe is continually speeding up and slowing down, controlled by an "advance" signal from a preview head which samples the signal level on the master tape two seconds (one full revolution of the disc) before the music gets to the cutting head.
When the record is played, the tone arm must also track inward toward the center at a continually varying rate. To facilitate this, pivoted arms are made with extremely low bearing friction so that the stylus, as it tracks the spiral groove, can easily carry the arm inward with it. A small handful of radial arms (particularly those which float on air-pressure bearings) also have low enough friction to follow the groove without aid. But most radial arms have far too much bearing friction to be moved by the slight sidethrust provided by the compliance of the stylus assembly, and so they must be driven inward by motors. Lacking a preview mechanism to measure the varying groove pitch, most radial arms depend on a servo system to detect a deviation of the arm from its desired tangency and generate motor drive signals which move the arm inward until tangency is restored.
Thus, the traditional goal of the radial arm-to mimic the cutting head in its perfect and constant tangency-is only a theoretical ideal. In reality most radial ("linear-tracking" or "straight-line tracking") arms have a designed in tangency error: The arm must deviate from perfect tangency in order for the servo to operate. Therefore, the tangency error is continually changing, departing from zero and being re-corrected when it becomes large enough to trigger the servo. The size of the error depends on the servo's response window: ±0.5° is typical. So, when we compare a radial design versus a pivoted arm, we are not matching an error-free system against one with angular errors; rather, each type of arm has its own characteristic pattern of tangency error.
This is illustrated in Fig. 1, which shows the tangency error of a radial arm with a 0.5° servo window versus that of a pivoted arm having an effective length of 9 inches, an offset angle of 24°, and an overhang of 18 mm. The vertical scale is actually the "tracking error index" in degrees/cm, obtained by dividing the tangency error (in degrees) by the disc radius (the distance of the stylus from the center spindle, in cm). The equations for computing this tracking index are given in References' 1 and 2. The innermost grooves on a typical 12-inch LP record are at a radius of 6 cm, while the outermost grooves are 14.5 cm from the center.
The distortion produced by lateral tracking error is proportional to this tracking index rather than to the actual tangency error in degrees. As Fig. 1 shows, the tracking index for a correctly designed 9-inch arm is only 0.13 deg/cm at worst and under 0.1 deg/ cm over most of the record surface. A radial arm with a servo window of 0.5° has a tracking index which varies from 0.04 deg/cm in the outer grooves to 0.08 deg/cm in the inner grooves.
But this discussion is still theoretical. In the real world, in order to have a tracking error index as low as that in Fig. 1 with either a pivoted or radial arm, you would have to be very lucky or very skillful, because in real tone arms these theoretical differences in tracking error are usually swamped by the inevitable small imperfections in the installation of the phono cartridge in the headshell. Ideally, the cartridge body should be exactly parallel to the headshell axis, but slight twists of one or two degrees of arc are fairly common-especially since many cartridges and headshells still are made with curved contours instead of the straight edges which would make it easier to judge parallelism by eye. Errors of a couple of millimeters in the longitudinal position of the stylus in the headshell are also fairly common, particularly in view of the lack of precision in the procedures supplied for setting overhang in many arms.
Even if you are an experienced and careful installer of cartridges in head shells, you probably can't be certain of achieving an accuracy better than 0.5° in alignment and about 1 mm in longitudinal position. (It may seem easy to set the longitudinal position in a radial arm, since the stylus should pass directly over the center of the spindle, but the spindle is 7.2 mm in diameter, so an error of only 14% of the spindle diameter still amounts to a full millimeter!) Figure 2 illustrates the tracking error index which these small errors will produce in a radial arm, individually and in combination; the dashed line shows the total tracking error when a servo wobble of 0.5° is added to cartridge alignment errors of 0.5° in angle and 1 mm in position.
For comparison, Fig. 3 shows how the tracking error index of the pivoted arm is affected by the same slight alignment errors. Unlike the radial arm, where positive and negative errors produce the same tracking distortion, the pivoted arm produces a more complex family of curves depending on the direction as well as the amount of the error. But it is clear that a tracking index of 0.2 deg/cm or greater can easily occur with both types of tone arm. As expected, the worst-case combinations of angular and linear shift in the pivoted arm yield larger amounts of tracking error in the pivoted arm than in the radial. But, remarkably, other combinations actually lead to lower tracking distortion in the pivoted arm than in the radial! In two of the curves in Fig. 3, the tracking error of the pivoted arm remains under 0.15 deg/cm over most of the disc surface.
At any rate, it seems clear that, under real-world operating conditions, radial arms can claim only a general tendency toward lower tangency error, not a clear-cut superiority. In fact, it can be argued that the design of the headshell is a more important contributor to accurate tangency than is the choice of radial or pivoted arm. A headshell that provides unambiguous seating of the cartridge without any possibility of twist, and is equipped with a precision jig for setting the stylus overhang, substantially improves the odds that the theoretically low tracking error of either type of arm will actually be realized in practice.
Of course, this doesn't mean that the question of tangency error in pivoted arms can simply be ignored. As Fig. 3 shows, the tracking error of a pivoted arm can be degraded by quite small geometrical errors, and many pivoted arms exhibit substantial amounts of tracking error because of incorrect geometric design-even though the equations for minimizing tracking error in pivoted arms have been available to engineers for 40 years. Therefore, when installing a cartridge in a pivoted arm, you can't guarantee minimum tracking error just by being careful. As a precaution you also should use an alignment protractor such as the DB Systems DBP-10 or the Dennesen Soundtrackor to check and correct any errors in the arm's geometric design. With such an aid, the real-world performance of a pivoted arm can come close to achieving the theoretical minimum tracking error shown in Fig. 1.
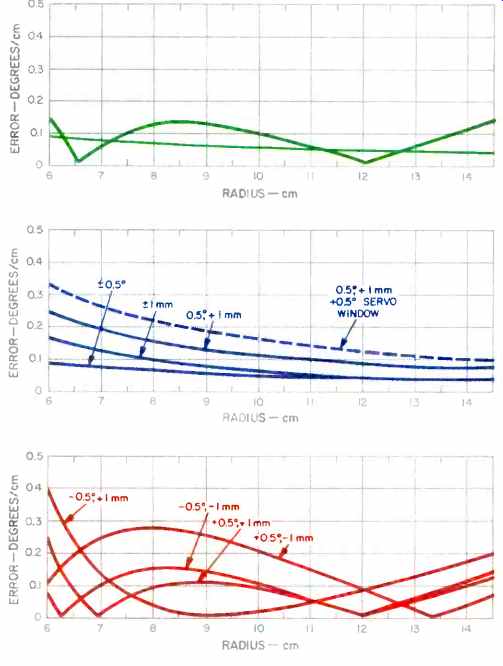
Fig. 1-Tracking error index of a radial arm, light fine, with a 0.5° servo
window and that of a pivoted arm, heavy line, with a 9 inch effective length,
24° offset angle, and 18-mm overhang.
Fig. 2-Tracking error index of a radial arm, showing how various settings influence the index.
Fig. 3-Tracking error index of a pivoted arm, showing how various settings influence the index.
Skating Force
If radial arms don't guarantee dramatically lower tangency error than pivoted arms, surely there is one area where their superiority is unquestioned: Perfect freedom from skating force. In radial arms the friction of the stylus in the groove produces a drag force which is directed along the length of the arm and so has no effect, but in pivoted arms the offset angle of the headshell transforms part of that drag force into a sidethrust which pulls the cartridge toward the center of the record. Modern tonearms are equipped with anti-skating devices which cancel this thrust by providing an outward force of approximately equal strength.
The catch is that the drag, and thus the sidethrust, is not constant. It varies with groove modulation, increasing during loud passages and decreasing during soft ones. At best, the anti-skating device can only compensate for the average (or highest) value of the sidethrust. With a pivoted arm the varying sidethrust will cause continual slight variations in the effective tracking force on the two groove walls.
Freedom from this, it is speculated, may be a significant area of superiority for the radial arm.
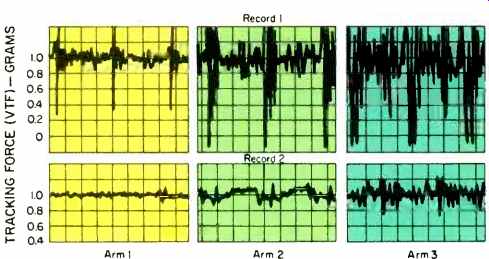
Fig. 4-Changes in vertical tracking force with changes in tonearm mass
and with warped and flat records; see text.
Such speculations, however, tend to ignore the actual values of the forces involved. The maximum level of the sidethrust, in a loud passage, is about 15% of the vertical tracking force. The minimum level in a soft passage is at least half of that value, i.e. 7% of the VTF. Thus, if we were to set the anti skating force to a median value (11% of the VTF), the uncompensated side thrust would only vary by ±4% of the VTF, i.e. 0.04 gram for a 1-gram VTF. Does anyone seriously believe that a 0.04-gram difference in the effective tracking force on the left and right groove walls could make any audible or measurable difference? If we adopt the common approach of setting the anti-skating for optimum tracking of the loudest passages, we are effectively compensating for the maximum sidethrust, and the forces on the left and right groove walls will become unbalanced by 7% or 8% of the VTF at low modulation levels. (That is, the force on the left-channel wall will decrease by 8% and the force on the right wall will increase by 8% for a total difference of 16%.) Could this have a detectable effect on stereo imaging or on the resolution of subtle details during low-level passages? Fortunately, this question can easily be answered by direct experiment, so you can decide it for yourself. Select a turntable whose anti-skating can easily be varied during play without disturbing the tonearm. Adjust the VTF and anti-skating for optimum tracking of loud passages, and then play an extended low-level passage of music.
While listening, reduce the anti-skating force to about half of its preset value (producing balanced forces on the two channels), and then raise it to normal (creating the unbalanced condition for low-level groove modulations). If you hear a clear difference in sound, you may be a candidate for a radial arm.
Most people, with most playback systems, hear little or no difference in this test.
While theory indicates that the varying sidethrust in a pivoted arm will cause variations of a few percent in the effective tracking force on each groove wall, it would be interesting to measure the dynamic forces on the stylus while actually playing a record and see if these sidethrust variations show up under real-world playing conditions. This experiment can be done, using a strain-gauge cartridge. The varying d.c. and infrasonic output from this type of cartridge is directly proportional to the deflection of the cantilever and thus to the instantaneous force bearing on the stylus.
Such a measurement was made a few years ago by Poul Ladegaard at Bruel & Kjaer, and Fig. 4 shows the result (reproduced from Ref. 3). The initial tracking force was set to 10 mN (1.0 gram), and portions of two records were played with the same cartridge mounted in three different tone arms. Each oscillogram in Fig. 4 shows the variation in effective tracking force during four seconds of music (two revolutions of the record). This result is shocking. Instead of the variations of a few percent which might be caused by skating forces, we see the tracking force varying by 20%, 50%, and even more. What, you might ask, is going on to produce these results?
Tonearm Mass and Resonance
The answer is that the three tone arms had differing values of effective mass, arm 1 being the lightest. Record 1 (the upper row of three oscillograms) had a medium-sized warp, while record 2 appeared flat to the eye. What Fig. 4 shows is that under real-world operating conditions, with arms of typical mass and records of typical warp content, the effective tracking force is continually varying-and by much more than a few hundredths of a gram attributable to sidethrust variation.
Note that with arm 3 (the heaviest of the three), tracking force variations of ±20% were found even when playing a record which looked perfectly flat.
With arm 2, a typical medium-mass design, the force still varies by ± 10% on a flat disc.
By itself, the tracking force is not important; as long as the net tracking force is high enough to keep the stylus in contact with the groove wall, it will continue to trace the groove. The real problem here is the varying deflection of the stylus cantilever, which is what is actually being measured in these graphs. With any operating cartridge the arm's tracking force bears down against the resilient compliance of the stylus assembly, and so any variation in force immediately produces a corresponding deflection of the cantilever (and vice versa). This has several deleterious effects:
1. As the height of the cartridge above the disc surface varies, the vertical tracking angle (VTA) and stylus rake angle (SRA) also vary, producing harmonic and IM distortion.
2. Up-and-down motion of the cartridge relative to the record surface produces a back-and-forth motion of the stylus along the groove, causing the music to be frequency-modulated.
This is a form of flutter, and it tends to occur at rates of a few Hertz-precisely the rates at which the ear is most sensitive to flutter. Experiments have demonstrated that, under typical operating conditions, turntables often exhibit higher amounts of wow and flutter than their specifications indicate, and this flutter is closely correlated with the frequency and severity of the arm/cartridge resonance. Taming the resonance reduces the flutter.
3. As the cartridge shakes, its tracking force is modulated up and down--the stylus alternately digging in with too much force (creating the possibility of accelerated record wear) and nearly floating free with insufficient force for undistorted tracking of the groove.
This is clearly seen in the upper row of Fig. 4; there are moments when the effective tracking force falls to zero, meaning that the stylus has completely lost contact with the groove. Of course, when the stylus is tracing heavily modulated grooves, a reduction of even 20% in effective tracking force may be enough to cause increased distortion and mistracking.
4. If the varying cantilever deflection is not purely up and down but involves any rotary or side-to-side motion, the stereo imaging will vary as well.
If you look closely at the oscillogram for arm 3, you will see that the wiggles are not random but regularly spaced, and analysis shows that the cantilever deflection is occurring at a rate of 7 Hz. This, of course, is no accident: The frequency of the arm/cartridge resonance (due to the arm's effective mass reacting with the pickup's compliance) is 7 Hz. If this frequency is substantially lower than about 9 or 10 Hz, the resonant system will continually be stimulated into oscillation by motor rumble and by record warps-not just the obvious ripples but also the smaller surface irregularities which infest virtually every disc. Moreover, in the real world the shelf on which the turntable sits is not motionless; it has its own resonances, stimulated by the infrasonic vibrations of street traffic, footfalls, refrigerator compressors, furnace blowers, etc. And since most turntable suspension systems become progressively less effective with decreasing frequency, these perturbations are readily transmitted to the record and the stylus.
There are two practical ways to stabilize the cantilever deflection. One is to use a damping mechanism such as the brush supplied with Shure and Stanton/Pickering cartridges, a DiscTraker or Zerostat Z-track device, the silicone damping supplied with some tonearms, or the resonance-cancelling rubber decoupler built into the counterweight of some arms. (The most ambitious of the decouplers is perhaps that in the Dual arms, where the device is "tunable" to the mass and compliance of the cartridge used.) The other approach is to keep the frequency of the arm/cartridge resonance above 8 Hz or so, where there will be fewer perturbations to stimulate it. In principle, this could be accomplished by lowering the cartridge's compliance, but the low-frequency tracking ability of any cartridge is proportional to its compliance, so a relatively high compliance is mandatory.
Therefore, the only way to raise the resonant frequency is to reduce the effective mass of the cartridge and arm. Figure 4 testifies to the effectiveness of this approach: The oscillograms for arm 1, the low-mass arm, show virtually no variation in cantilever deflection on the flat record (lower curve) and almost instantaneous recovery from perturbations on a warped disc (upper curve). It may seem that I have wandered away from the subject of this article, which is a comparison of pivoted and radial tonearms, but I really haven't.
What I want to suggest is that the most important advantage of radial arms is simply that they are lighter than most pivoted arms. Being lighter, they are typically freer of the many problems described in the preceding paragraphs-resonance-induced flutter, varying VTA and SRA, blurred stereo imaging, and mistracking due to variations in effective tracking force. Being lighter, they approach closer to the tonearm ideal: Providing a stable platform for the cartridge and holding it at a fixed distance above the record for stable cantilever deflection and constant tracking force.
Radial arms are typically lighter than pivoted arms because they are shorter and simpler. As S. K. Pramanik explained in Ref. 2, the effective mass of the tonearm is mainly due to the cartridge and plug-in headshell-the items farthest from the tonearm's pivots. And their effective mass varies with the square of their distance from the pivots. A typical pivoted arm is about 9 inches long, while a typical radial arm is only 6.5 inches long; squaring these numbers, we get 81 and 42.25, indicating that when all else is equal, the effective mass of the radial arm is only half that of its pivoted counterpart.
Of course, the same reduction in effective mass can also be achieved in a pivoted arm--as Dual, SME, and Ortofon have successfully demonstrated--by redesigning the headshell, plug-in socket, and cartridge itself, halving the weight of each. The collaboration between Ortofon and Dual, for instance, has resulted in a tonearm/cartridge system whose total effective mass is only 8 grams.
Thus, when audiophiles graduate from older pivoted arms to radial arms and discover that they sound better, what they are hearing is probably not the presumed superiority of straightline tracking, nor a reduction in tangency error or skating force. They are simply hearing the benefits of a low mass tonearm and cartridge combination in practice.
If this conclusion is correct, then the main virtue of radial arms is just a lucky accident which may well have had nothing to do with the designer's original goal! It is intriguing to note that when audiophiles discuss the observed advantages of radial arms, they tend to describe the very same benefits that other listeners have observed when switching to a low-mass arm (and the same benefits which. other audiophiles have reported when damping was added to control the resonance of a tonearm): Better tracking, lower distortion, less flutter, improved stereo imaging, better resolution of inner detail, etc.
Conclusion
The average radial arm probably does sound better than the average pivoted tonearm because the radial arm, being shorter, has lower inertia and thus provides stable cantilever deflection and constant tracking force.
But those pivoted arms which have been designed for radically lowered mass also exhibit these same virtues--and are fully the equal of radials in sonic performance. Since the weight of the cartridge body is a major contribution to the effective mass of a tone arm, it is fortunate that a growing variety of low-mass cartridges are becoming available.
The influences of arm mass and resonance on the quality of reproduced sound are well established and easily demonstrated. The advantages of straight-line tracking appear to be more theoretical than real.
References
1. Kessler, Martin D. and B. V. Pisha, "Tonearm Geometry and Setup Demystified," Audio, January 1980, p. 76; "Addenda," Audio, April 1980, p. 26.
2. Pramanik, S. K., "Understanding Tonearms," Audio, June 1980, p. 52.
3. Ladegaard, Poul, "Audible Effects of Mechanical Resonances in Turntables," B&K Application Note 17-233, 1978.
4. Foster, A., et al., "BAS Turntable Clinic," Boston Audio Society Speaker, 8:4 and 8:5, January and February 1980.
(Source: Audio magazine, June 1982)
Also see:
Linear Tracking Turntables (June 1981)